Free Falling
Build a Drop Tower to experimentally determine the gravitational constant.

Lesson plan
1. Prepare
- Read through the student material in the EV3 Classroom App.
- Collect some information about the law of falling bodies.
- If you don’t have double-block class time, plan to run this lesson over multiple sessions.
Part A
2. Engage (10 Min.)
- Use the ideas in the Ignite a Discussion section below to engage your students in a discussion related to this lesson.
- Split your class into pairs.
3. Explore (35 Min.)
- Have each pair of students build the Drop Tower.
- Give them some time to do a test run to ensure that the model is built correctly and works as expected.
Part B
4. Explain (15 Min.)
- Have each team perform the experiment at least three times, and record their results.
- Make sure they can create their own testing tables.
5. Elaborate (30 Min.)
- Have your students use their recorded data to calculate the average velocity and the gravitational constant using the law of falling bodies.
- Ask each team to briefly summarize the results of their experiments.
- Don’t forget to leave some time for cleanup.
6. Evaluate
- Give feedback on each student’s performance.
- You can use the assessment rubrics provided to simplify the process.
Ignite a Discussion
Free fall describes the acceleration of an object due exclusively to gravity. People who jump out of an airplane are slowed by aerodynamic resistance as they fall. A true free fall would only be possible in a vacuum in which no forces other than gravity are able to act. A test environment for experiments of this kind can be found at NASA’s Glenn Research Center in Cleveland, Ohio.

Start a discussion about free falling by asking relevant questions, like:
- What does the term free fall refer to?
- What’s the relation between acceleration and velocity?
- In a vacuum, does a hippopotamus fall at exactly the same rate as an earthworm?
- When can knowledge about the concept of free fall come in handy?
Building Tips
Building Instructions
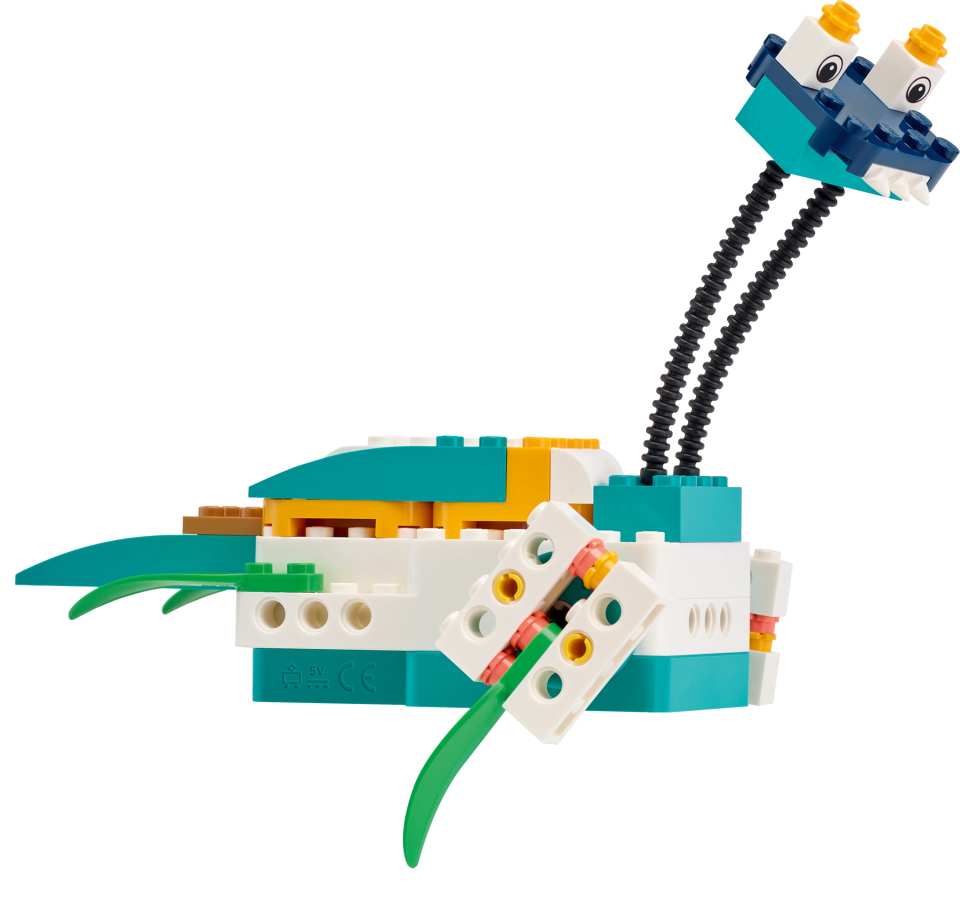
Using the Model
Place the model on a solid and level surface. Run the program and wait until the Touch Sensor is shown on the Display. Place the ball in the ball arm and press the Touch Sensor on the back of the Drop Tower to start a drop experiment. If the experiment was successful, a big smile followed by the fall time will be shown on the Display. If not, a sad face will be shown. The ball arm closes automatically for the next experiment.
Running the Experiment
As they’re running their experiments, remind your students of the following:
- If the experiment was successful, the fall time will be shown on the Display.
- Record the experiment number and fall time in a testing table. Make sure to leave enough space for additional columns for further calculations.
- Perform the experiment at least three times and use the average value to ensure the most reliable results.
Coding Tips
Program

Differentiation
Simplify this lesson by:
- Working with your students to solve some of the calculations
Take this lesson to the next level by:
- Encouraging your students to perform additional calculations using the measured values
- Explaining the significance of average values (e.g., arithmetic mean versus the median, susceptibility to outliers) that can be used to equalize measurement errors across a series of experiments
- Challenging the students to come up with ways of improving the accuracy of their experiments
Assessment Opportunities
Teacher Observation Checklist
Create a scale that matches your needs, for example:
- Partially accomplished
- Fully accomplished
- Overachieved
Use the following success criteria to evaluate your students’ progress:
- Students applied mathematical concepts and/or processes to determine the gravitational constant.
- Students explained that the ball accelerates at a constant rate.
- Students identified the independent and controlled variables of their experiments.
Self-Assessment
Have each student choose the level that they feel best represents their performance.
- Bronze: I’ve performed the experiments but didn’t calculate the average velocity or gravitational constant.
- Silver: With some help, I’ve calculated the average velocity and gravitational constant.
- Gold: I’ve calculated the average velocity and gravitational constant.
- Platinum: I’ve not only calculated the average velocity and gravitational constant, but I’ve also performed additional calculations using the measured values.
Language Arts Extension
To integrate language arts skills development, have your students:
- Create a brief report focusing on the result of their experiment and how it compares with the accepted value for g, the acceleration of gravity near the surface of Earth
- Create a presentation explaining the results of their experiment and what they’ve learned
Note: This will make for a longer lesson.
Career Links
Students who enjoyed this lesson might be interested in exploring these career pathways:
- Manufacturing and Engineering (Pre-Engineering)
- Science, Technology, Engineering & Mathematics (Science and Math)
Teacher Support
Students will:
- Learn to determine the gravitational constant
- Learn that there are laws of gravitation that apply to all objects
NGSS
MS-PS2-2
Plan an investigation to provide evidence that the change in an object’s motion depends on the sum of the forces on the object and the mass of the object.
Common Core
CCSS.ELA-LITERACY.RST.6-8.3
Follow precisely a multistep procedure when carrying out experiments, taking measurements, or performing technical tasks.
CCSS.ELA-LITERACY.RST.6-8.4
Determine the meaning of symbols, key terms, and other domain-specific words and phrases as they are used in a specific scientific or technical context relevant to grades 6-8 texts and topics.
CCSS.ELA-LITERACY.WHST.6-8.7
Conduct short research projects to answer a question (including a self-generated question), drawing on several sources and generating additional related, focused questions that allow for multiple avenues of exploration.
CCSS.MATH.CONTENT.8.EE.A.2
Use square root and cube root symbols to represent solutions to equations of the form x² = p and x³ = p, where p is a positive rational number. Evaluate square roots of small perfect squares and cube roots of small perfect cubes. Know that √2 is irrational.
ISTE
5B
Students collect data or identify relevant data sets, use digital tools to analyze them, and represent data in various ways to facilitate problem-solving and decision-making.